Visuelle Konzepte     Tanz der Teiler D     Dance of the Divisors E

(Klick to magnify)

The dance ends with the number, which is divisible by all basic numbers from 1 to 9.

It started with the fact that I thought about the number 12. This number seems meaningful to me, but also old-fashioned-medieval: 12 inches equal one foot, at the market you buy a dozen eggs and so on.

Perhaps, I speculate, to favour the dozen over the ten is probably because the dozen contains more divisors than the ten. You can divide 12 by 2, 3, 4, and 6, while 10 is divisible only by 2 and 5, provided we live in the world of natural numbers.

The most fundamental principle of intellectual thinking is duality––thinking in pairs of opposites––which we find in science, philosophy and religion. (e.g., plus/minus, good and evil, on/off, finite/infinite.) The finite is easy to recognize – as an antithesis we conclude the infinite that eludes our imagination. Many decision-making processes begin with yes or no. The Yin-Yang symbol represents duality. The principle of thesis and antithesis, each of which carries a grain of its opposite within. From thesis and antithesis, synthesis can be deduced. This brings us to the number 3. For the three, there are quite a few mythical references. For example, "Father, Son and Holy Spirit" (the Holy Trinity).

The doubling of 2 and 3 gives 4 and 6. The I Ching - (Chinese oracle) is built on this principle. 1 (odd) results in a continuous line, 2 (even) results in a broken line. A finished symbol consists of 2 groups of 3 superimposed lines (= 6 lines).

This logic, which builds on 2 and 3 leads us back to the number 12.

Assuming that the benefit of a dozen lies in its high divisibility, I wondered which is the first number divisible by all the basic numbers from 1 to 9. This number can be considered as the opposite of a prime number (which contains no divisors except––what is inevitable––1 and itself).

That would be the search for the "anti-prime-figure".

You can easily look up something like that on the Internet today. But I felt like tackling the solution of this problem without formulas, using a visual system.

So I thought up the following experiment:

To create a diagram on whose vertical axis (y) the divisors are 1 to 10 and on the horizontal axis (x) the numbers from 1 to that which is finally divisible by all basic numbers.

If the number we are looking for is divisible by all basic numbers, divisors 2 and 5 necessarily occur. Thus I know that the number we want must end with a zero, and therefore must be divisible by 10. This means it is sufficient to use the numbers in steps of ten.

The non-mathematical procedure:

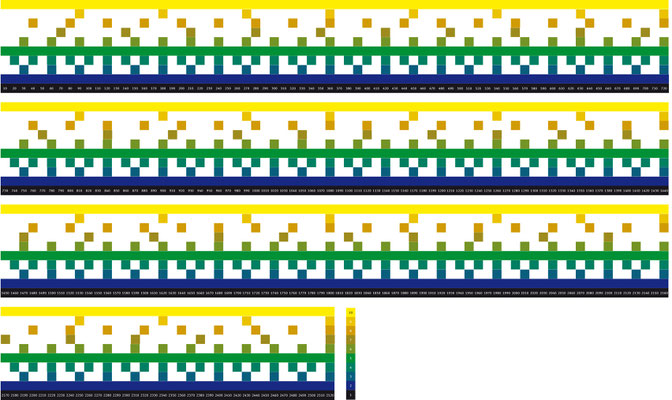
Basically, I need 10 basic elements, of which each represents a divisor. So, what is required for 1 a piece of 1x1 units, for 2 a piece of 2x1 units, for 3 accordingly 3x1 units and so on. The last square (of 1x1 units) on each element is coloured (a different colour for each divisor). If I arrange enough of these parts logically, I will eventually come to a column on which all divisors occur. I now know that this is the case at 2520.

I also know, since I am only using the numbers of the series of ten, that lines of the 1, 2, 5 and 10 divisors result in continuous bars. What I also discovered while experimenting is that the pattern (in the 10-divisor configuration) repeats after 36 columns––except for the number 7*. This opens up fundamentally new possibilities for tackling the graphic implementation.

But I would like to dwell on the procedure with sections for the time being. In order to arrive at a representation by manual means, instead of the columns that represent the individual numbers, I can create strips that each represent a particular divisor.

Let's start laconically with divisor 1: All numbers are divisible by 1. This results in a continuous bar. This also applies to divisor 2, 5 and 10, because in our experiment we use only multiples of tens.

For the divider 3 I can make strips on which every 3rd position is coloured. Similarly, divider 4 is coloured on every 2nd position. In the same way we proceed to divisors 6 and 8 where each 3rd or 4th position is coloured. With numbers 7 and 9, only the 7th and 9th positions are coloured.

*To conclude our experiment we need 7 "packages" containing 36 columns. Maybe this has to do with the fact that only the row of divisor 7 shifts, compared to all other numbers that are positioned identically in each of these packages.

That could look like this: